The reader will observe, that throughout the chapter to which this note refers, as well as in the note itself, the argument of Hume is taken strictly according to his own interpretation of the terms he uses, and the calculations are founded on them; so that it is from the very argument itself, when fairly pursued to its full extent, that the refutation results.
Both our belief in the truth of human testimony, and our belief in the permanence of the laws of nature, are, according to Hume, founded on experience; we may, therefore, in the complete ignorance in which he assumes we are, with respect to the causes of either, treat the question as one of the probability of an event deduced solely from observations of the past. [192/193]
The argument of Hume asserts, that one improbability, namely, that of the falsehood of the testimony in favour of a miracle, must always be greater than another improbability, namely, that of the occurrence of the miracle itself; and also, that, from the very nature of human experience, this preponderance can never take place.
Now the only possible mode of disproving the assertion, that one thing cannot, under any circumstances, be greater than another, is to measure, under all circumstances, the numerical value of the two things so compared, and the truth or falsehood of the assertion will then appear. The doctrine of chances, which has been much improved since the time of Hume, now enables us to apply precise measures to this argument; and it is the object of this Note to state the outlines of the calculation, and the results to which it leads. Previously to this, however, it may not be amiss to offer a few remarks on the principles about to be employed.
In the great work of Laplace, "Théorie Analytique des Probabilités," those principles are established, and they are not merely undisputed, but are admitted by other writers of the highest authority on this subject. They form a part of the received knowledge of the present day, and, as such, they are employed in the present work, in which I propose to use, not to [193/194] discuss them. I state this, because it has occasionally been asserted by persons unacquainted with the doctrine of chances, that the argument respecting the probability or improbability of miracles does not admit of the application of numbers. The received foundations of science are not to be put aside by such opinions, however highly skilled their authors may be in other branches of knowledge, and however powerful the intellect by which they may have attained those acquirements. The conclusions arrived at by the application of pure analysis must ever rest on the truth of the principles assumed at the commencement of the inquiry; and although a knowledge of mathematics may not appear necessary for forming a right judgment of the accuracy of those principles, yet it is observed, that a clear apprehension of them is not often found in the minds of those who are unacquainted with that science. When, however, the grounds on which the principles employed in the doctrine of chances are called in question by competent authority, it will be time enough to examine the question; and none will more eagerly enter upon that examination than those best versed in it, for none are so well aware of the extreme difficulty and delicacy of the subject.
As confusion sometimes arises from the difference in the meaning of the words probable and improbable in popular language and in mathematical inquiries, [194/195] it may be convenient to point it out; and to state, that in this Note it is used in the mathematical sense, unless the reader's attention is directly called to a question relating to its popular sense.
In common language, an event is said to be probable when it is more likely to happen than to fail: it is said to be improbable when it is more likely to fail than to happen.
Now, an event whose probability is, in mathematical language 1/p, will be called probable or improbable, in ordinary language, according as p is less or greater than 2.
If, in mathematical language, 1/p expresses the probability of an event happening, 1-1/pp expresses the probability of its failing, or the improbability of its happening.
It has been stated in the text, that two views may be taken of those extraordinary deviations from the usual course of nature, called miracles. According to the first of these, we have to calculate the probability that a white ball has been drawn from an urn (containing only white and black balls, out of which m balls have been drawn all black), as deduced from [195/196] the testimony of witnesses whose probability of speaking truth is known: — or, of the analogous case; it having been observed that m persons have died without any restoration to life, what is the probability that such a resurrection has happened, it having been asserted by n independent witnesses, the probability of each of whose speaking false is -1/p ?
The probability of the death without resurrection of the m + 1th, and the improbability of such an occurrence, independently of testimony, is 1/m+2; which is therefore the probability of a contrary occurrence, or that of a person being raised from the dead.
Now only two hypotheses can be formed, collusion being, by hypothesis, out of the question: either the event did happen, and the witnesses agree in speaking the truth, the probability

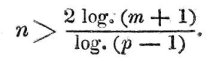
If p is any number greater than two, this equation can always be satisfied.
It follows, therefore, that, however large m may be, or however great the quantity of experience against the occurrence of a miracle, (provided only that there are persons whose statements are more frequently correct than incorrect, and who give their testimony in favour of it without collusion,) a certain number n Can ALWAYS be found; so that it shall be a greater improbability that their unanimous statement shall be a falsehood than that the miracle shall have occurred. [197/198]
Let us now suppose each witness to state one falsehood for every ten truths p = 11, and m = 1000,000,000,000;
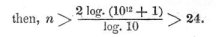
or twenty-five such- witnesses are sufficient.
If the witnesses only state one falsehood for every hundred truths, then thirteen such witnesses are sufficient.
Another view of the question might be taken; and it might be asserted that, in order to believe in the miracle, the pro- bability of its truth must be greater than the probability of its falsehood; in this case the expression (A) must be greater than (B) or,


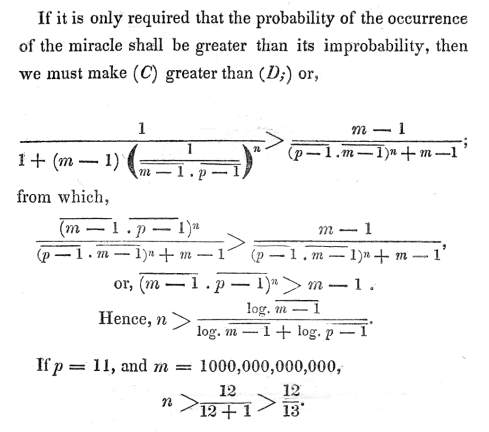
Hence in this view, also, a sufficient number of witnesses of given veracity may always be found to render the improbability of their concurrent independent testimony being false, greater than the improbability of the occurrence of the miracle. There is, however, one other view, which it seems probable would have been that taken by Hume himself, had he applied numbers to his own argument. Considering the probability of the coincidence in falsehood of « persons each having the probability [2201/02]
in favour of his truth, which is — u, that probability ought to be less than that of the occurrence of the miracle; or,

This view of the question refers to the probability of the concurrence of the witnesses before they have given their testimony. The other four cases relate to the probability of the miracle having happened, as deduced from the fact of the testimony having been given. The last seems to have been that which Hume would have himself arrived at; the others represent the true methods of estimating the probabilities of the various cases: and the important conclusion follows, that, whichever be the interpretation given to the argument of Hume, if independent witnesses can be found, who speak truth more frequently than falsehood, it is always possible to assign a number of independent witnesses, the improbability of the falsehood of whose concurring testimony shall be greater than that of the improbability of the miracle itself. [202/203]
It is to be observed, that the whole of this argument applies to independent witnesses. The possibility of the collusion, and the degree of credit to be assigned to witnesses under any given circumstances, depend on facts which have not yet been sufficiently collected to become the subject of mathematical inquiry. Some of those considerations which bear on this part of the subject, the reader will find treated of in the work of Dr. Conyers Middleton, entitled " A Free Inquiry into the Miraculous Powers which are supposed to have subsisted in the Christian Church, from the earliest Ages through several successive Centuries." London, 1749. [203/204]